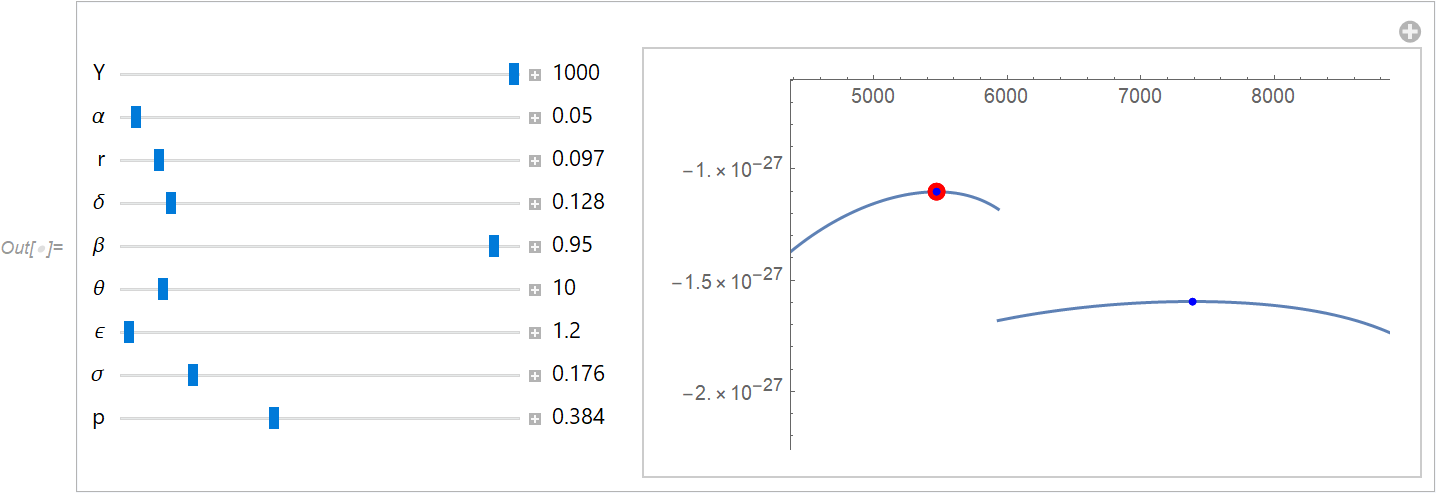
As a check on varying parameter values consider some sort of a Manipulate:
Manipulate[
sol1 = Maximize[{f1, H <= (Y - Y δ)/(r + α),
H > 0, (Y - r*H) ⩾ 0}, H];
sol2 = Maximize[{f2, H > (Y - Y δ)/(r + α),
H > 0, (Y - r*H) ⩾ 0}, H];
max = If[sol1[[1]] > sol2[[1]], sol1, sol2];
hmin = 0.8 H /. sol1[[2]];
hmax = 1.2 H /. sol2[[2]];
{ymin, ymax} =
MinMax[{f1 /. H -> hmin, f1 /. H -> (Y - Y δ)/(r + α),
f2 /. H -> (Y - Y δ)/(r + α), f2 /. H -> hmax}];
y0 = ymin - 0.95 (ymax - ymin);
y1 = ymax + 1.05 (ymax - ymin);
Show[Plot[f1, {H, hmin, (Y - Y δ)/(r + α)}, PlotRange -> {{hmin, hmax}, {y0, y1}}],
Plot[f2, {H, (Y - Y δ)/(r + α), hmax}, PlotRange -> {{hmin, hmax}, {y0, y1}}],
ListPlot[{{{H /. max[[2]], max[[1]]}}, {{H /. sol1[[2]], sol1[[1]]}},
{{H /. sol2[[2]], sol2[[1]]}}}, PlotStyle -> {{PointSize[0.03], Red}, Blue, Blue}]],
{{Y, 1000}, 1, 1000, Appearance -> "Labeled"},
{{α, 0.05}, 0.02, 1, Appearance -> "Labeled"},
{{r, 0.05}, 0.01, 1, Appearance -> "Labeled"},
{{δ, 0.3}, 0.01, 1, Appearance -> "Labeled"},
{{β, 0.95}, 0, 1, Appearance -> "Labeled"},
{{θ, 10}, 0, 100, Appearance -> "Labeled"},
{{ϵ, 1.2}, 0, 100, Appearance -> "Labeled"},
{{σ, 0.2}, 0, 1, Appearance -> "Labeled"},
{{p, 0.51}, 0, 1, Appearance -> "Labeled"},
TrackedSymbols :> {Y, β, θ, α, r, ϵ, σ, δ, p},
Initialization :> (f1 = ((1 + (1 - p) β) ((-H r + Y)^(1 - 1/ϵ) + (H θ)^(1 - 1/ϵ))^((1 - 1/σ)/
(1 - 1/ϵ)))/(1 - 1/σ) + (p β ((Y - H (r + α))^(1 - 1/ϵ) +
(H θ)^(1 - 1/ϵ))^((1 - 1/σ)/(1 - 1/ϵ)))/(1 - 1/σ);
f2 = (p β ((Y δ)^(1 - 1/ϵ) + 5000^(1 - 1/ϵ) θ^(1 - 1/ϵ))^((1 - 1/σ)/
(1 - 1/ϵ)))/(1 - 1/σ) + ((1 + (1 - p) β) ((-H r + Y)^(1 - 1/ϵ) +
(H θ)^(1 - 1/ϵ))^((1 - 1/σ)/(1 - 1/ϵ)))/(1 - 1/σ))]
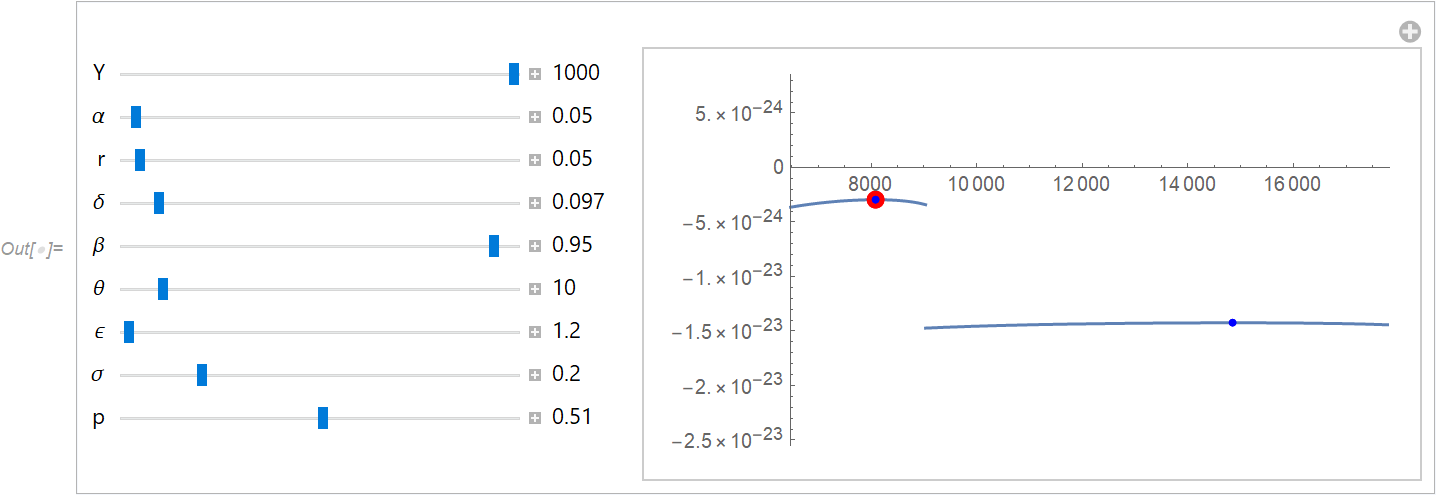
Note that it appears that $\beta$, $\theta$, $\epsilon$, $\sigma$, and $p$ have no effect on either part of the function.