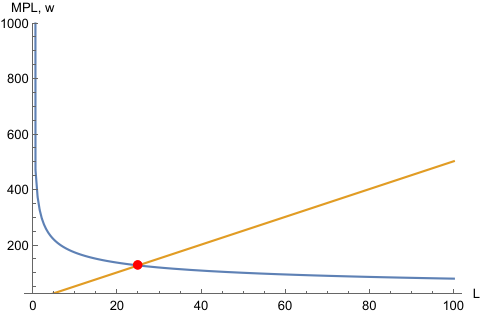
Mesh and MeshFunctions is work.
Here we write a MeshFunctions such as
MeshFunctions -> Function[l, mpl[a, k, l, d] - lsupply[b, m, l]]
and set the Mesh->{{0}} indicate that mpl[a, k, l, d] - lsupply[b, m, l]==0,so we need not solve the equation by hand.
Clear["Global`*"]
y[a_, k_, l_, d_] = a*k^d*l^(1 - d);
mpl[a_, k_, l_, d_] = (1 - d)*a*k^d*l^(-d);
lsupply[b_, m_, l_] = b + m*l;
Manipulate[
Plot[{mpl[a, k, l, d], lsupply[b, m, l]}, {l, 0, 100},
PlotRange -> {25, 1000}, AxesLabel -> {"L", "MPL, w"},
LabelStyle -> Black, Mesh -> {{0}},
MeshStyle -> Directive[PointSize[Large], Red],
MeshFunctions ->
Function[l, mpl[a, k, l, d] - lsupply[b, m, l]]], {{a, 80, "A"}, 1,
100}, {{k, 350, "K"}, 200, 1000}, {{d, 1/3}, 10^-2, 1}, {{b, 4}, 0,
200}, {{m, 5}, 0, 10}]