Skip to main content
Mathematica

Return to Question

Notice removed Canonical answer required by CommunityBot
Bounty Ended with no winning answer by CommunityBot
Notice added Canonical answer required by mathemania
Bounty Started worth 50 reputation by mathemania
Made an update
Source Link
mathemania
mathemania
  • 725
  • 5
  • 12

UPDATE:

I noticed that when I changed the lower limit of the integral in intS I can get the same curve but with different magnitude so I think that cannot be correct.

intS[a_?NumericQ, z_?NumericQ] := NIntegrate[(1/x^d) (1/((1 - (x/zh)^(d + 1)) (1 - cz[a, z]^2 x^(2 d))))^(1/2), {x, 10^-2, z}, MaxRecursion -> 20, PrecisionGoal -> 6, Method -> {"GlobalAdaptive"}]

pic1

intS[a_?NumericQ, z_?NumericQ] := NIntegrate[(1/x^d) (1/((1 - (x/zh)^(d + 1)) (1 - cz[a, z]^2 x^(2 d))))^(1/2), {x, 1, z}, MaxRecursion -> 20, PrecisionGoal -> 6, Method -> {"GlobalAdaptive"}]

pic2

You can see that when I changed the lower limit to 10^-2 the curve already appeared but the magnitude is wrong, and when I set it to 1 the curve is there and the magnitude is closer to the original. However, I think I cannot just tune the lower limit, it must be the case that $\epsilon \leq z_s \leq z_h$ since $\epsilon$ is a regulator. Note that since that is the case, $z_s$ should approach $\epsilon$ first then take the limit where $\epsilon \rightarrow 0$. So in the case of Mathematica I can just set $\epsilon$ to the machine-wise zero, I think $10^{-10}$ is considered to be 0 but not really setting 0.

UPDATE:

I noticed that when I changed the lower limit of the integral in intS I can get the same curve but with different magnitude so I think that cannot be correct.

intS[a_?NumericQ, z_?NumericQ] := NIntegrate[(1/x^d) (1/((1 - (x/zh)^(d + 1)) (1 - cz[a, z]^2 x^(2 d))))^(1/2), {x, 10^-2, z}, MaxRecursion -> 20, PrecisionGoal -> 6, Method -> {"GlobalAdaptive"}]

pic1

intS[a_?NumericQ, z_?NumericQ] := NIntegrate[(1/x^d) (1/((1 - (x/zh)^(d + 1)) (1 - cz[a, z]^2 x^(2 d))))^(1/2), {x, 1, z}, MaxRecursion -> 20, PrecisionGoal -> 6, Method -> {"GlobalAdaptive"}]

pic2

You can see that when I changed the lower limit to 10^-2 the curve already appeared but the magnitude is wrong, and when I set it to 1 the curve is there and the magnitude is closer to the original. However, I think I cannot just tune the lower limit, it must be the case that $\epsilon \leq z_s \leq z_h$ since $\epsilon$ is a regulator. Note that since that is the case, $z_s$ should approach $\epsilon$ first then take the limit where $\epsilon \rightarrow 0$. So in the case of Mathematica I can just set $\epsilon$ to the machine-wise zero, I think $10^{-10}$ is considered to be 0 but not really setting 0.

Minor correction
Source Link
mathemania
mathemania
  • 725
  • 5
  • 12

where $c=c(z_s)$ and $a$ is a function of $z_s$, and $a$, $z_h$ are constantsis a constant which can be given a value later. Also, even though $a$ is a function of $z_s$ in the expression, in the final expression for $S$ I will just give it a value. $d$ is just a dimension parameter which can be given a value say, $d=3$.

where $c=c(z_s)$ is a function of $z_s$, and $a$, $z_h$ are constants which can be given a value later. $d$ is just a dimension parameter which can be given a value say, $d=3$.

where $c=c(z_s)$ and $a$ is a function of $z_s$, and $z_h$ is a constant which can be given a value later. Also, even though $a$ is a function of $z_s$ in the expression, in the final expression for $S$ I will just give it a value. $d$ is just a dimension parameter which can be given a value say, $d=3$.

Source Link
mathemania
mathemania
  • 725
  • 5
  • 12

Eliminate a variable within an integral

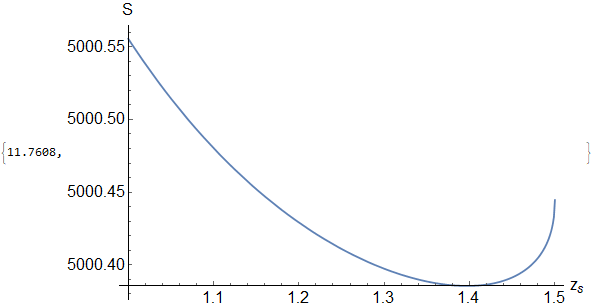
I have two expressions given by,

$$a = \int_0^1 dx \frac{c z_s^{d+1} x^d}{\sqrt{(1-(z_s/z_h)^{d+1} x^{d+1})(1-c^2 z_s^{2d} x^{2d})}} \tag{1}\label{1},$$

$$S = \int_\epsilon^{z_s} dx \frac{1}{x^d} \sqrt{\frac{1}{(1-(x/z_h)^{d+1})(1-c^2 x^{2d})}}\tag{2}\label{2},$$

where $c=c(z_s)$ is a function of $z_s$, and $a$, $z_h$ are constants which can be given a value later. $d$ is just a dimension parameter which can be given a value say, $d=3$.

The goal is to find an expression of $S$ such that $c$ is eliminated. Now, one condition which can help achieve that is,

$$\frac{dS}{dz_s} = 0\tag{3}\label{3},$$

actually I need to find an expression for the minimal $S$ (with respect to $z_s$) to which $c$ dictates.

I have asked some related problems before but for eq. $\eqref{2}$ in those cases I made a transformation $x=z_sy$ so that the integral limits there is $[\epsilon/z_s,1]$ and then expand out the finite and divergent terms then I just showed the finite terms there so that I allow the lower limit to be 0.

Solving a function inside an integral

Smoothen the result of FindRoot

In this case, I just start with the original integral $\eqref{2}$ and set a small number for $\epsilon$ (this is just a modification of my previous problem in order to eliminate the divergence contribution but the result should not differ for those regions far from the divergence region).

The problem is when I try to plot those same quantities I get a different result.

The previous code,

d = 3;
zh = 1.5;
torootL[a_, c_?NumericQ, z_] := a - NIntegrate[(c z^(d + 1) xd^(d/(d + 1)))/((1 - xd (z/zh)^(d + 1)) (1 - xd^((2 d)/(d + 1)) c^2 (z)^(2 d)))^(1/2), {xd, 0, 1}, MaxRecursion -> 12, PrecisionGoal -> 6, Method -> {"GlobalAdaptive", "SingularityHandler" -> "DoubleExponential"}]
cz[a_?NumericQ, z_?NumericQ] := c /. FindRoot[torootL[a, c, z], {c, 0.001, 0.0000001, 1000}]
intS[a_?NumericQ, z_?NumericQ] := NIntegrate[With[{b = z/zh}, (((-1)/(d - 1)) cz[a, z]^2 z^(2 d)) x^d ((1 - (b x)^(d + 1))/(1 - cz[a, z]^2 (z x)^(2 d)))^(1/2) - ((b^(d + 1) (d + 1))/(2 (d - 1))) x ((1 - cz[a, z]^2 (z x)^(2 d))/(1 - (b x)^(d + 1)))^(1/2) + (b^(d + 1) x)/((1 - (b x)^(d + 1)) (1 - cz[a, z]^2 (z x)^(2 d)))^(1/2)], {x, 0, 1}, MaxRecursion -> 12, PrecisionGoal -> 6, Method -> {"GlobalAdaptive", "SingularityHandler" -> "DoubleExponential"}]
functionS[a_, z_] = ((-((1 - cz[a, z]^2 z^(2 d)) (1 - (z/zh)^(d + 1)))^(1/2)/(d - 1)) + intS[a, z] + 1)/(4 z^(d - 1));
Plot[functionS[0.1, z], {z, 1, zh}, PlotPoints -> 8, AxesLabel -> {"z_s", "S"}, AxesStyle -> Directive[Black, 18], PlotStyle -> Thick, PlotRange -> Full, ImageSize -> Large] // Quiet // AbsoluteTiming

produces this plot,

pic1

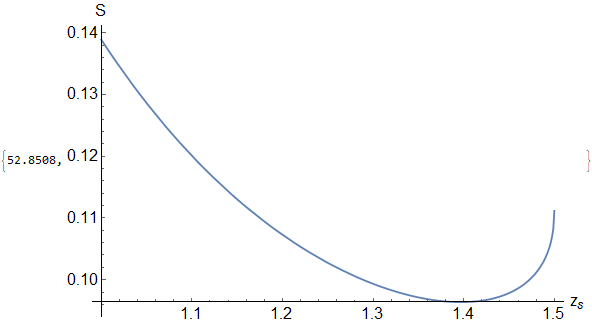
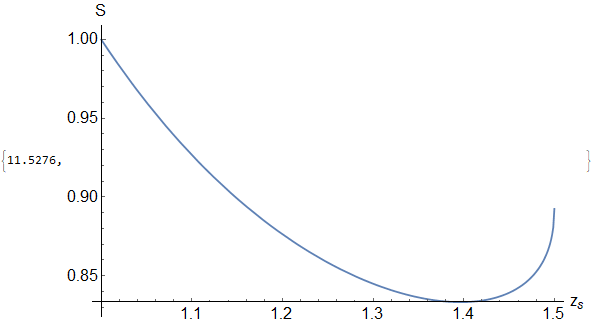
The present code where I just changed the lines corresponding to the discussion I made above,

d = 3;
zh = 1.5;
torootL[a_, c_?NumericQ, z_] := a - NIntegrate[(c z^(d + 1) xd^(d/(d + 1)))/((1 - xd (z/zh)^(d + 1)) (1 - xd^((2 d)/(d + 1)) c^2 (z)^(2 d)))^(1/2), {xd, 0, 1}, MaxRecursion -> 12, PrecisionGoal -> 6, Method -> {"GlobalAdaptive", "SingularityHandler" -> "DoubleExponential"}]
cz[a_?NumericQ, z_?NumericQ] := c /. FindRoot[torootL[a, c, z], {c, 0.001, 0.0000001, 1000}]

intS[a_?NumericQ, z_?NumericQ] := NIntegrate[(1/x^d) (1/((1 - (x/zh)^(d + 1)) (1 - cz[a, z]^2 x^(2 d))))^(1/2), {x, 10^-10, z}, MaxRecursion -> 20, PrecisionGoal -> 6, Method -> {"GlobalAdaptive"}]
functionS[a_, z_] = intS[a, z] + 1/(z^(d - 1));
Plot[functionS[0.1, z], {z, 1, zh}, PlotPoints -> 8, AxesLabel -> {"z_s", "S"}, AxesStyle -> Directive[Black, 18], PlotStyle -> Thick, PlotRange -> Automatic, ImageSize -> Large] // Quiet // AbsoluteTiming

produces the plot,

pic2

where the Method I used in the present is only GlobalAdaptive since if I use the Method in the old code it produces a flat line which I don't know why. Anyways, the old and present codes should produce the same plot especially in those region close to $z_s = 1.5$ to which in the first image you can see a minimum occurring around $z_s \approx 1.4$.

Why is this so? I'm still trying to understand the NIntegrate Integration Strategies documentation although it is hard to understand for me since I'm not a heavy user maybe until now.

I was thinking of maybe there is a different strategy to eliminate $c$ in the expression for $S$ using $\eqref{1}$ $\eqref{2}$ $\eqref{3}$ at the same time. Any comments?