You can use .5 {-y, x}/(x^2 + y^2) // TraditionalForm to see that .5 {-y, x}/(x^2 + y^2) can be use to express the vector value function.
$$\left(\frac{-0.5y}{x^2+y^2},\frac{0.5x}{x^2+y^2}\right)$$
and actually,{-y, x}/(x^2 + y^2) is the gradient of the function ArcTan[y/x] or ArcTan[x,y]
Grad[ArcTan[x, y], {x, y}]
Grad[ArcTan[y/x], {x, y}] // Simplify
(* {-(y/(x^2 + y^2)), x/(x^2 + y^2)} *)
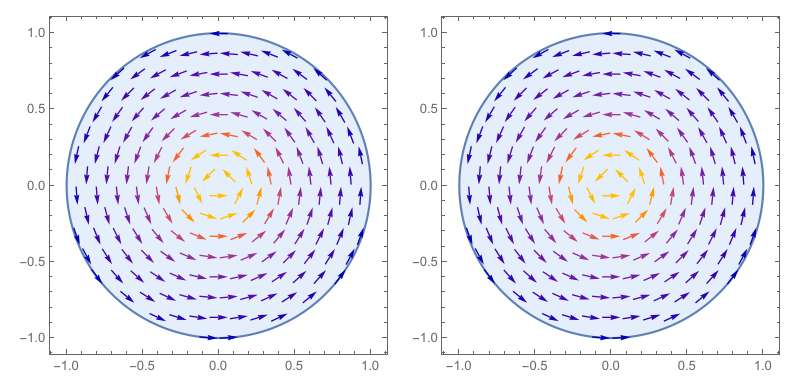
GraphicsRow[{VectorPlot[{-(y/(x^2 + y^2)), x/(
x^2 + y^2)}, {x, y} \[Element]∈ Disk[]],
VectorPlot[
Grad[ArcTan[x, y], {x, y}] // Evaluate, {x, y} \[Element]∈ Disk[]]}]