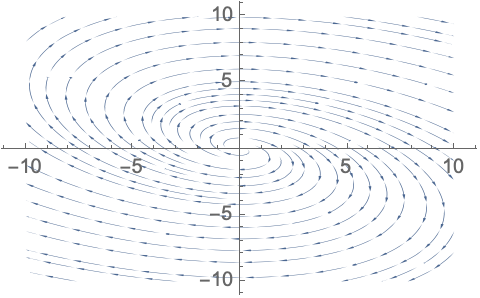
Since we're not given an ODE by the OP, it's hard to comment on which phase portrait is correct. But taking the OP's desire for clockwise flow as given and working backwards, we might reach the following system (or a positive multiple of it): $${dx \over dt} = x+2y,\ {dy \over dt} = -x \,.\tag{1}$$ In that case, the phase field is given by
Clear[f]
f[x_, y_] := {x + 2 y, -x};
StreamPlot[f[x, y], {x, -10, 10}, {y, -10, 10}, Frame -> False,
Axes -> True, AspectRatio -> 1/GoldenRatio, StreamStyle -> "PinDart"]
(If the ODE/system was ${d^2y\over dt^2} + {dy \over dt} + 2y=0$, with $x = dy/dt$, then the flow as plotted would be the same as above, because the "position" y and the "velocity" x are switched from the usual velocity versus position. If the ODE/system was $dy/dx = x/(-x-2y)$, then $dx/dt = 1 > 0$ and all trajectories move to the right; the original stream plot was correct. And if the ODE/stytemsystem was a negative multiple of (1), then the phase flow is counterclockwise; this is also the case if the ODE was ${d^2y\over dt^2} + {dy \over dt} + 2y=0$, with $x = dy/dt$counterclockwise.)