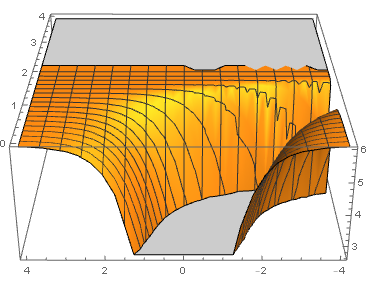
I have the following solution of the SDE: $$U(x,t)=-6+12 * \tanh \left[x+\left(B(t)-\frac{t^{2}}{2}\right)+\int_{0}^{t} e^{s^{2}} d s\right]^{2}$$
Where $B(t)$ is white noise.
In the following code and Figure 1, the solution is drawn without noise
Plot3D[-6 + 12*Tanh[x + (0 - t^2/2) + \!\(
\*SubsuperscriptBox[\(\[Integral]\), \(0\), \(t\)]\(
\*SuperscriptBox[\(E\),
SuperscriptBox[\(s\), \(2\)]] \[DifferentialD]s\)\)]^2, {t, 0,
4}, {x, -4, 4}]
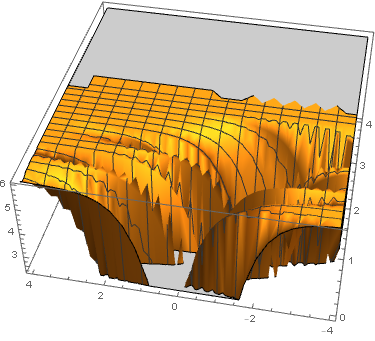
In the following code and Figure 2, the solution is drawn with noise
tmax = 10;
samplesPerSec = 10;
\[Omega]ω = 2;
\[Sigma]σ = 1;
noise = Interpolation[
Normal[RandomFunction[
WhiteNoiseProcess[\[Sigma]]WhiteNoiseProcess[σ], {0, tmax*samplesPerSec}]][[1]]];
Plot3D[-6 + 12*Tanh[x + (noise[t*samplesPerSec] - t^2/2) + \!\(
\*SubsuperscriptBox[\(\[Integral]\∫\), \(0\), \(t\)]\(
\*SuperscriptBox[\(E\),
SuperscriptBox[\(s\), \(2\)]] \[DifferentialD]s\)\)]^2, {t, 0,
4}, {x, -4, 4}]
The question is how can I get a figure that contains more noise as in Figure 3
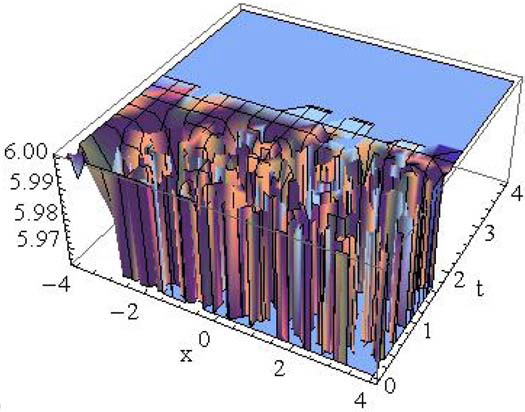
Is there a better way to add more Noise to the solution and draw it?