I have data from similar function that are not evaluated on the same points. I would like to plot the points and the curves that are the upper and lower bounds of them. As an example consider the following code:
f[x_, r1_, r2_] := 1/((x + (2 r1 - 1)/2)^2 + r2 + 1)
SeedRandom[3]
points = {};
rs = Table[{RandomReal[], RandomReal[]}, {20}];
Do[
p = RandomReal[{-2, 2}, {50}];
v = f[#, rs[[i, 1]], rs[[i, 2]]] & /@ p;
points = Join[points, Transpose[{p, v}]];
, {i, 20}]
points = RandomSample[points];
r2 = RandomReal[];
v = f[#, r1, r2] & /@ p;
points = Join[points, Transpose[{p, v}]];
, {20}]
points = RandomSample[points];
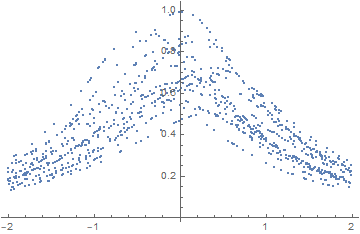
The ListPlot of these points is:
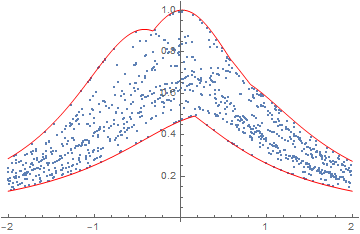
Clearly, in this example since we know the functions that create the points it is easy to get the upper and lower bounds:
But if we know only the points, is there any easy way to get these curves (or some others that are relatively close to those)?