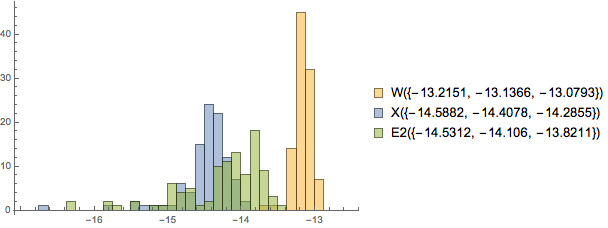The following is somewhat faster. The principal changes are:

• The use of a distance function Sqrt@Total[(#1-Transpose@#2)^2]& that computes an array of distances for disfun[x, {y1, y2,...}] that is much faster than mapping Norm over individual pairs.

• The use of Dot instead of Sum. Dot is much faster. In fact, the uncompiled function, which is fully vectorized, is sometimes faster than the compiled function.

• The vectorized use of RBF to compute the final Φ, following @xzczd's example.

• The use of LinearSolve instead of Inverse, which is both faster and more numerically stable. (The maximum relative difference in the solutions was about 10^-13 to 10^-12 on a few random examples.)

Since not all distance functions and RBFs can be vectorized, some tests were added to switch to the slower point-by-point methods when the faster methods are not possible.

Clear[RBFInterpolationE2]

Options[RBFInterpolationE2] = {
   "DistanceFunction" -> Automatic,(*(Sqrt@Total[(#1-Transpose@#2)^2]&)=Norm[x1,#]&/@x2*)
   "RadialBasisFunction" -> (Sqrt[#1^2 + #2^2/4] &),
   "RadialScale" -> Automatic, "Debug" -> False, "Compile" -> False};

RBFInterpolationE2[cptab_, opts : OptionsPattern[]] := 
  Module[{ro, xpts, fundata, Φ, disfun, λ, RBF, x, dfThreadableQ, rbfListableQ, body},
   xpts = #[[1]] & /@ cptab;
   fundata = #[[2]] & /@ cptab;
   disfun = OptionValue["DistanceFunction"];
   If[disfun === Automatic,
    disfun = Sqrt@Total[(#1 - Transpose@#2)^2] &; (* vectorized & "threadable" norm *)
    dfThreadableQ = True,
    dfThreadableQ = False  (* could add options or heuristics *)
    ];
   
   RBF = OptionValue["RadialBasisFunction"];
   rbfListableQ = ListQ[RBF[{0.}, 1.]];
   
   If[dfThreadableQ,
    (*Φ=DistanceMatrix[xpts] (* not faster for the default distance *)*)
    Φ = disfun[#, xpts] & /@ xpts,
    Φ = Table[disfun[xpts[[i]], xpts(*[[j]]*)], {i, 1, Length[xpts]}, {j, 1, Length[xpts]}]
    ];
   Which[
    OptionValue["RadialScale"] == Automatic
    , ro = Median[Flatten[Table[Drop[Φ[[i]], {i}], {i, 1, Length[Φ]}]]],
    NumberQ[OptionValue["RadialScale"]]
    , ro = OptionValue["RadialScale"],
    True
    , Print["I cannot understand \"RadialScale\"->", 
     OptionValue["RadialScale"], " So I'm going to make it up"]
    ; ro = Median[Flatten[Table[Drop[Φ[[i]], {i}], {i, 1, Length[Φ]}]]]
    ];
   If[rbfListableQ,
    Φ = RBF[Φ, ro],   (*xzczd; assumes RBF is Listable *)
    Φ = Map[RBF[#, ro] &, Φ, {2}]
    ];
   
   λ = LinearSolve[Φ, fundata]; (* was λ=Inverse[Φ].fundata *)
   
   With[{λi = λ, xptsi = xpts, roi = ro, RBFi = RBF, disfuni = disfun},
    If[dfThreadableQ, (* construct code for the interpolating function *)
     body = Hold[x, Dot[λi, RBFi[disfuni[x, xptsi], roi]]],
     body = Hold[x, Dot[λi, RBFi[disfuni[x, #] & /@ xptsi], roi]]
     ];
    If[OptionValue["Compile"],
     Return[body /. Hold[x_, code_] :>
        Compile[{{x, _Real, 1}}, code,
         RuntimeAttributes -> {Listable}, Parallelization -> True]],
     Return[body /. Hold[x_, code_] :> Function[x, code]]
     ]]
   ];

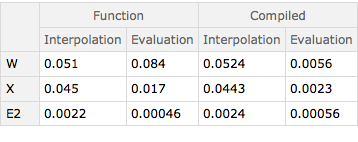
The following shows the timings of Mr.Wizards, xzczd's and my codes.

ClearAll[run];
run[meth_String] := Module[{ans, funFun},
   With[{RBFI = ToExpression["RBFInterpolation" <> meth]},
    <|
     "Function" -> <| 
       "Interpolation" -> First@RepeatedTiming[funFun = RBFI[cptab, "Compile" -> False]],
       "Evaluation" -> First@RepeatedTiming[ans = funFun /@ xpts],
       "RBFI" -> funFun,
       "Values" -> ans
       |>,
     "Compiled" -> <| 
       "Interpolation" -> First@RepeatedTiming[funFun = RBFI[cptab, "Compile" -> True]],
       "Evaluation" -> First@RepeatedTiming[ans = funFun /@ xpts],
       "RBFI" -> funFun,
       "Values" -> ans
       |>
     |>
    ]];


ds = Dataset[AssociationMap[run, {"W", "X", "E2"}]];
(* kinda roundabout transposing, maybe *)
Transpose[ds[[All, All, {"Interpolation", "Evaluation"}]]][[All, All, All]] // Transpose

The parallelization of the compiled function is not used in the above timings. If we use parallelization (by calling the compiled function on all points at once), the compiled function beats the uncompiled one (Mac, Intel i7, 4(8) cores):

funFun = ds["E2", "Compiled", "RBFI"];
First@RepeatedTiming[funFun@xpts]
(*  0.00014  *)

The OP compares the three methods with the OP's original code at points between the interpolation points. All three methods do pretty well at these points.

With[{errdata = 
   Reap[Query[All, "Compiled", Sow@RealExponent[#Values - fundata] &]@ds][[2, 1]]},
 Histogram[
  MapThread[Legended[#, #2@Quartiles[#]] &, {errdata, Normal@Keys[ds]}],
  {1./8}, PlotRange -> {{-17, -12.5}, All}]
 ]

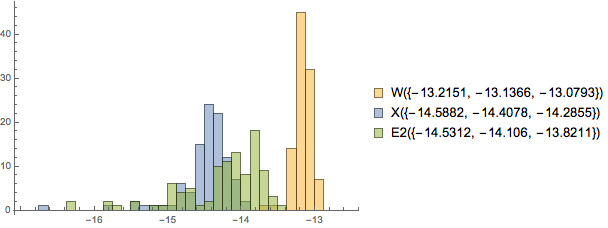
The following compares the three methods with the OP's original code at points between the interpolation points. Mr.Wizard s code is exactly equal to the OP's. There's a small but significant error in xzczd's results, which I do not have time to explore. The error in my results are consistent with the differences in the code, such as LinearSolve instead of Inverse (the condition number of Φ is around 10^5 or so on the random point sets cptab I checked).

funFun = RBFInterpolation[cptab, "Compile" -> True];
valsOP = funFun /@ MovingAverage[xpts, 2]; 
cfs = Query[All, "Compiled", "RBFI"]@ds // Normal // Values;
errdata2 = (Transpose[Through[cfs[#]] & /@ MovingAverage[xpts, 2] - valsOP]);

Grid@Transpose@{Normal@Keys[ds], Quartiles /@ RealExponent@errdata2}