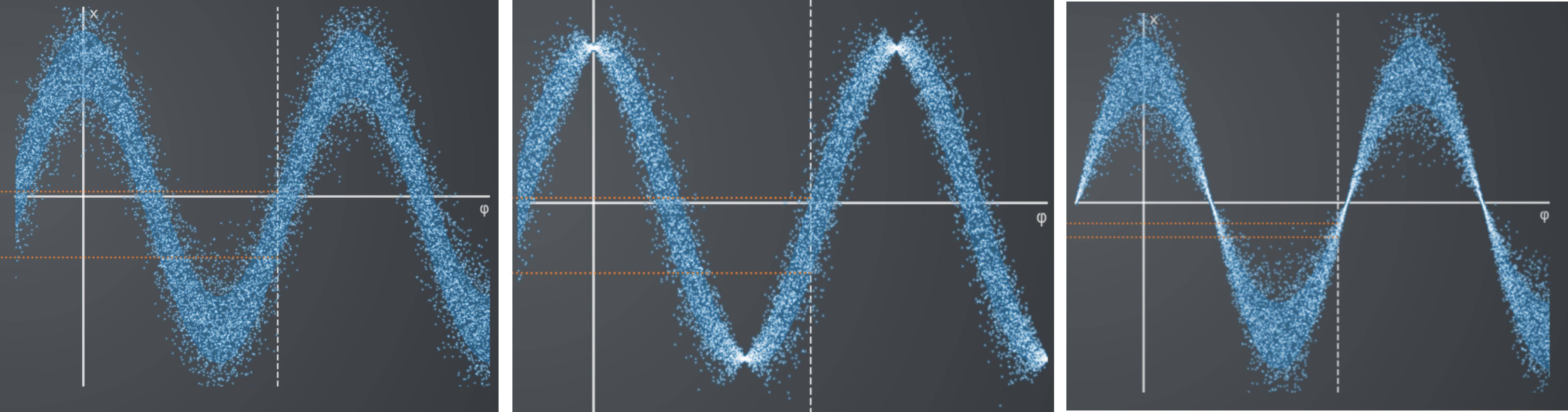
I would like to generate data in Mathematica that follows a sinusoidal curve. However, I would like to add different types of noise to the curves so as to reproduce graphs similar this:

I have checked posts related to adding noise to data but am still unsure on how to do this. Any help would be appreciated!

I would like to generate data in Mathematica that follows a sinusoidal curve. However, I would like to add a Gaussian noise to the curves so as to reproduce graphs similar this:

I have checked posts related to adding noise to data but am still unsure on how to do this. Any help would be appreciated!

CONTEXT These graphs represent the intensity-phase uncertainties of different quantum states. The first corresponds to the same uncertainty for both the number (amplitude of coherent states) and phase uncertainties, whilst the following two on the right depicts squeezing in one variable and an increased uncertainty in the other conjugate variable in a manner that obeys the Heisenberg uncertainty principle.

Although coherent states (laser light) have a Poissonian distribution in their photon time arrivals, the distribution of the electric field with varying phases will be Gaussian distributed.