I am using some extreme value fitting method which results in a parametric distribution for values exceeding some threshold, all values $\geq 0$.
For smaller values I'd like to use a smooth kernel distribution.
Pasting these two together, there is usually a small gap or jump in the distribution. So I looked for the possibility to 'fix' a point in the smoothed distribution, but found no such thing. Is there any way 'force' the smooth kernel distribution density to take a right end value?
It doesn't make a big difference, but there is simply no real reason for such a jump in the density.
Update: Of course it is possible to shift the empirical smoothed density and then scale the completeempirical density part to obtain a continuous density, but I am not really convinced. To me, it does not seem like the optimal solution. I'll attach two plots to illustrate the problem.
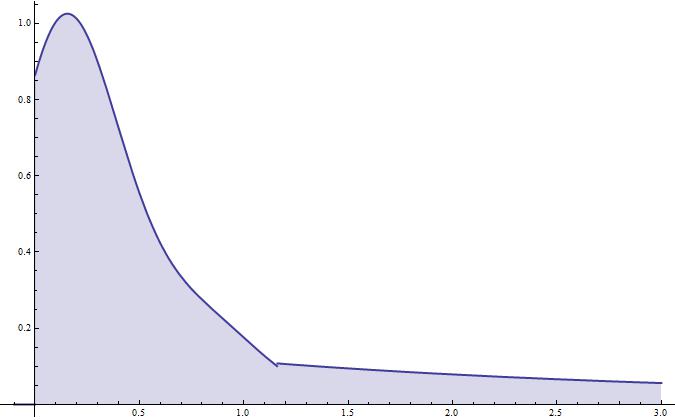
 Thank you!
Thank you!
