I have an exerciceexercise to linearize the following nonlinear system:
$$\dot{x} = A - B x - x y^2$$
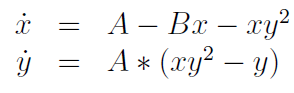 $$\dot{y} = A\cdot (x y^2 - y)$$
$$\dot{y} = A\cdot (x y^2 - y)$$
I tried it with osz = NonlinearStateSpaceModel[{{A - B*x - x*y^2, A*(x*y^2 - y)}, {x, y}}, {x, y}, {A, B}] for
osz = NonlinearStateSpaceModel[{{A - B*x - x*y^2, A*(x*y^2 - y)}, {x, y}}, {x, y}, {A, B}]
for the input of this system.
How can I linearize this system now?
StateTransformationLinearize[osz]
doesnt obviously work, because its not an affine system...
