If you solve for the second derivatives, you won't have to use "EquationSimplification" -> "Residual" and things will work ok.
Solving for the second derivatives be faster if you start with exact coefficients. Also, if you clear l, solve for the derivatives, and then substitute a value for l, Solve won't choke on the algebra. The long time it takes is probably why NDSolve advises the use of the option:
NDSolve::ntdv: Cannot solve to find an explicit formula for the derivatives. Consider using the option Method->{"EquationSimplification"->"Residual"}. >>
l(*l = 0.6 (1 + 0.00 I) // Rationalize;Rationalize;*) (* old solution *)
Clear[l];
g = 9.8 // Rationalize;
x0 = 0.01 // Rationalize;
(*... rest of OP's code...*)
(* This replaces eq[] in NDSolve; saves the result for ease of reuse *)
Clear[neweq];
neweq[n_, ω0_] := neweq[n, ω0] =
Equal @@@ First@ Simplify@
Solve[eq[n] /. ω -> ω0, Table[Subscript[x, i]''[t], {i, n}]]
(* Here is where we substitute the value for l *)
Clear[SOL];
SOL[n_] := NDSolve[{neweq[n, 1] /. l -> 0.6 (1 + 0.01 I), ic[n]},
Table[Subscript[x, i][t], {i, n}], {t, 0, 100}]
mysol = First@SOL[4]
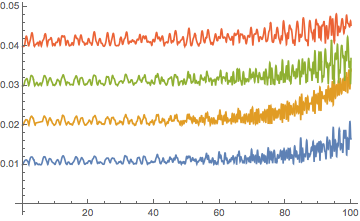
TheSince the solutions are complex, one has to decide what to plot. I chose the magnitude. The plots nearly overlap, so I offset them:
Plot[
0.01 Range[n] + Table[Subscript[xTable[Abs@ Subscript[x, i][t], {i, n}] /. mysol // Evaluate,
{t, 0, 100}]