UPDATE
In version 13.0.0 the described long-standing bug in determining PlotRange via AbsoluteOptions is fixed.
Original answer
AbsoluteOptions is known as very buggy function and the bug in determining the true PlotRange has very long history...
You could try my Ticks-based workaround for getting the complete PlotRange (with PlotRangePadding added):
completePlotRange[plot:(_Graphics|_Graphics3D|_Graph)] :=
Last@
Last@Reap[
Rasterize[
Show[plot, Axes -> True, Frame -> False, Ticks -> ((Sow[{##}]; Automatic) &),
DisplayFunction -> Identity, ImageSize -> 0], ImageResolution -> 1]]
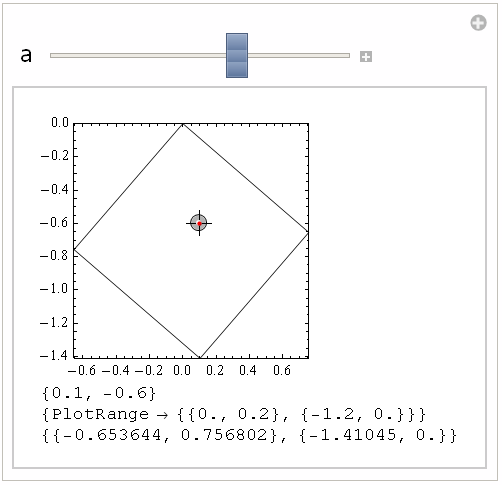
Manipulate[
DynamicModule[{pic},
Column[{pic =
Graphics[{FaceForm[], EdgeForm[Black],
GeometricTransformation[Rectangle[], RotationTransform[a]],
Red, Point[p]}, Frame -> True, PlotRangePadding -> 0], p,
AbsoluteOptions[pic, PlotRange], completePlotRange[pic]}]], {{a,
4}, 0, 2 Pi}, {{p, {.1, -.6}}, {-2, -2}, {2, 2}, Locator},
ContinuousAction -> False, SynchronousUpdating -> False]

EDIT
One can get the exact PlotRange (without the PlotRangePadding added) with the following function:
plotRange[plot : (_Graphics | _Graphics3D | _Graph)] :=
Last@
Last@Reap[
Rasterize[
Show[plot, PlotRangePadding -> None, Axes -> True, Frame -> False,
Ticks -> ((Sow[{##}]; Automatic) &), DisplayFunction -> Identity, ImageSize -> 0],
ImageResolution -> 1]]
Manipulate[
DynamicModule[{pic},
Column[{pic =
Graphics[{FaceForm[], EdgeForm[Black],
GeometricTransformation[Rectangle[], RotationTransform[a]],
Red, Point[p]}, Frame -> True], p,
AbsoluteOptions[pic, PlotRange], plotRange[pic]}]], {{a, 4}, 0,
2 Pi}, {{p, {.1, -.6}}, {-2, -2}, {2, 2}, Locator},
SynchronousUpdating -> False]
EDIT 2
Here is timing comparison of various ways to get real PlotRange:
completePlotRange[plot : (_Graphics | _Graphics3D | _Graph)] :=
Last@
Last@Reap[
Rasterize[
Show[plot, Axes -> True, Frame -> False, Ticks -> ((Sow[{##}]; Automatic) &),
DisplayFunction -> Identity, ImageSize -> 0], ImageResolution -> 1]]
completePlotRange[plot : (_Graphics | _Graphics3D | _Graph), format_] :=
Last@
Last@Reap[
ExportString[
Show[plot, Axes -> True, Frame -> False, Ticks -> ((Sow[{##}]; Automatic) &),
DisplayFunction -> Identity, ImageSize -> 0], format]]
pic = Graphics[{FaceForm[], EdgeForm[Black],
GeometricTransformation[Rectangle[], RotationTransform[.3]]},
Frame -> True];
Print[{#,
AbsoluteTiming[
First@Table[
completePlotRange[pic, #], {100}]]}] & /@ {"RawBitmap", "BMP",
"WMF", "EMF", "SVG", "PDF", "EPS"};
{RawBitmap,{2.8931655,{{-0.32158,0.981396},{-0.0250171,1.27587}}}}
{BMP,{3.0201728,{{-0.32158,0.981396},{-0.0250171,1.27587}}}}
{WMF,{4.3242473,{{-0.32158,0.981396},{-0.0250171,1.27587}}}}
{EMF,{4.0182298,{{-0.32158,0.981396},{-0.0250171,1.27587}}}}
{SVG,{3.1461800,{{-0.32158,0.981396},{-0.0250171,1.27587}}}}
{PDF,{16.9799712,{{-0.32158,0.981396},{-0.0250171,1.27587}}}}
{EPS,{7.3074179,{{-0.32158,0.981396},{-0.0250171,1.27587}}}}
AbsoluteTiming[First@Table[completePlotRange[pic], {100}]]
{2.3991372, {{-0.32158, 0.981396}, {-0.0250171, 1.27587}}}
One can see that Rasterize with ImageSize -> 0 is the fastest.
UPDATE 3
Here is purely Dynamic implementation of the same idea:
plotRange[plot : (_Graphics | _Graphics3D | _Graph)] :=
Reap[NotebookDelete[
First@{PrintTemporary[
Show[plot, Axes -> True, Frame -> False,
Ticks -> ((Sow[{##}]; Automatic) &),
DisplayFunction -> Identity, PlotRangePadding -> None,
ImageSize -> 0]], FinishDynamic[]}]][[2, 1]]
completePlotRange[plot : (_Graphics | _Graphics3D | _Graph)] :=
Reap[NotebookDelete[
First@{PrintTemporary[
Show[plot, Axes -> True, Frame -> False,
Ticks -> ((Sow[{##}]; Automatic) &),
DisplayFunction -> Identity, ImageSize -> 0]],
FinishDynamic[]}]][[2, 1]]
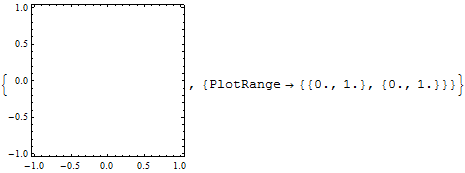




AbsoluteOptions$\endgroup$AbsoluteOptionsorGeometricTransformation. After all the latter one has records too. $\endgroup$GeometricTransformationis relevant here? In my understanding, the problem boils down to this simple example which returns wrong values:g = Graphics[{}, Frame -> True]; Print[g]; AbsoluteOptions[g, PlotRange]. I hope you don't mind that I added this to your Q to make it more general, if so, please feel free to revert. $\endgroup$